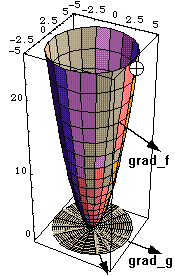
In Lecture 4 we discussed the gradient function. In this lecture we discuss an alternate method of solving some line integrals through the use of two new concepts - divergence and curl.
First, an elaboration on last lecture:
Two functions, interpreted in terms of a surface:
z=g(x,y)=x^2+y^2-C
f(x,y,z)=x^2+y^2-z=C

The gradient of f is perpendicular to the surface.
The curves in the x-y plane are for g=constant.
The gradient of g is a projection of the gradient of f onto the
x-y plane.
Let us relate certain line integrals to area integrals.
Remember the equations for flux and circulation:
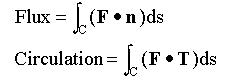
We will write these in a new way. Imagine a small chunk of the path
of length ds. From this, we find a new way of expressing T and n.
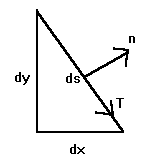
T=dx/dsi+dy/dsj
n=dy/dsi-dx/dsj
If F=Mi + Nj, then we can conclude:
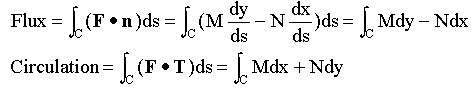
These equations are leading to divergence and curl.
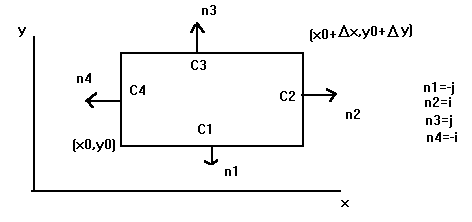
We will calculate the flux out of the box to find an expression for divergence.
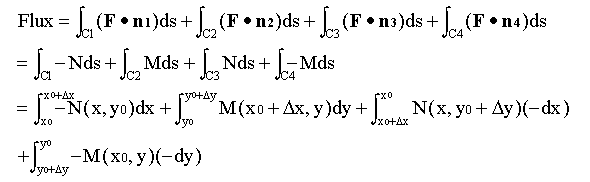
We continue by simplifying these integrals and then grouping N terms and
M terms together.
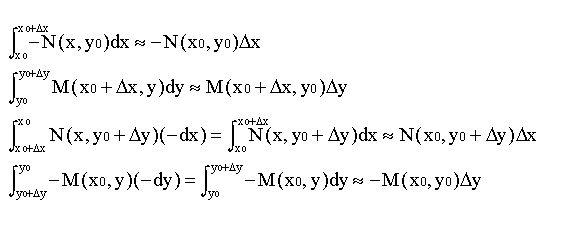
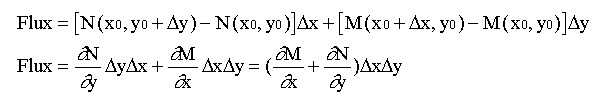
We see that the flux equals the area of the box times an expession of M and N.
This expression is defined as the divergence. The notation used to show
divergence
is
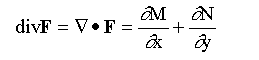
Also note that divF=flux/area.
In terms of fluid flow, the divergence can be interpreted as the flux over an
infinitely small loop.
We neglect to show the entire process for circulation, but through a
similar method to
the one shown above, it can be demonstrated that:
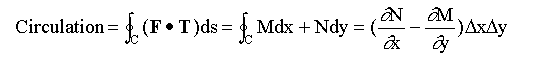
Once again, our result is the area of the box times a (somewhat different)
expression
of M and N. This expression is defined as the curl.
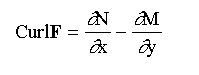
Note that the curl is equivalent to the circulation/area.
Also, curl is a vector quantity, but in 2 dimensions, this can be neglected
since the
direction of the vector will always be perpendicular to the 2 dimensions.
A more complete
definition will be given later in the course.
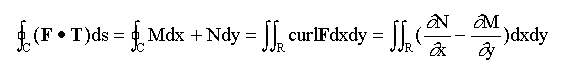
For flux:
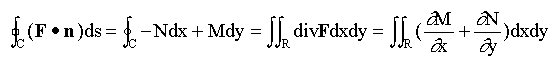
R is the region enclosed by C.
Green's Theorem converts the line integral into an area integral, which is
often easier to solve.
Examples will be given next lecture.