We covered the scalar dot product of two vectors in the last lecture and now move on to the second vector product that can be performed, the Cross Product. The Cross Product involves taking two vectors and getting as a result another vector which is perpindicular to both vectors. We use the formula below.

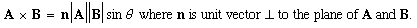
The direction of n follows the right hand rule, which is easy to learn. Tkae the picture below. Point your thumb in the direction of A, the first vector in the cross product. Now point your fingers in the direction of B. Your palm will face in the direction of n, out of the screen.

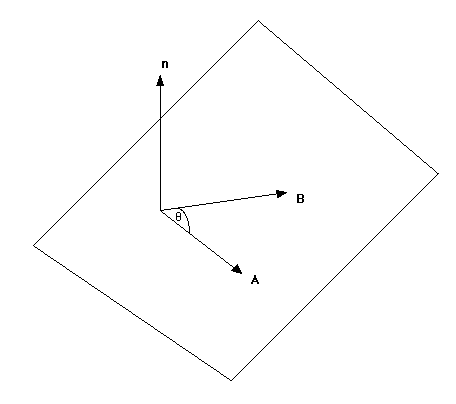
By this method we can do the Cross Products of i,j, and k.

ixj = k
jxk = i
kxi = j

NOTE: MOST RIGHT HANDED PEOPLE TEND TO LOOK AT THEIR LEFT HAND DURING TESTS, AS THEY'RE BUSY WRITING WITH THE RIGHT HAND. MAKE SURE TO USE THE RIGHT HAND, BECAUSE THE LEFT HAND WILL GIVE THE WRONG ANSWER.

• Motivations for the Cross Product.

  Mechanics- Torque

  

  To find the torque of a force, you multiply the magnitude of the force times the magnitude of the torque arm times the sine of the angle between them. The you define a vector in the direction a right handed screw would turn if twisted in the direction the force is being applied. That is exactly the same as the mathematics of the Cross Product.

  Area of a Parrallelogram

  

• Computing the Cross Product

  • By Distributive Property

    Example:
    A = i - j
    B = i + k
    AxB = (i - j) x (i + k) = ixi + ixk - jxi - jxk = (0 + (-j ) - (-k) - i)
    = -i - j + k

  • By Determinant Formula

    

  Note that both ways return the same answer. (This is very good.)

  ---

• Applications

  1. Find the Area of the shaded triangle.

     The area can be found using the fact that the area of the corresponding parrallelogram is |PAxPB|. So, the area of the triangle is one half of the area of the paralleogram, or .5*|PAxPB|.

     PA = -i + j
     PB = -i + j + k
     Area = .5*|PAxPB|
     = .5*|i + j| = sqrt(2)/2
     

  2. Find a vector perpendicular to triangle PAB.

     PAxPB = i + j

• 3D: Lines, Planes and Segments

  1. Lines

     

     v = Ai + Bj + Ck

     

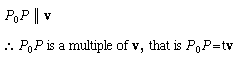
     (x-x0)i + (y-y0)j + (z-z0)k = t(Ai + Bj + Ck)
     Taking each component seperately
     x = x0 + tA
     y= y0 + tB
     z = z0 + tC
     

     Example:
     Find a vector parallel to the line described by the following equations
     x = 2 - t
     y= 3 + t
     z = 1 - 2t
     The Components of the vector are just the coefficients on t, so
     v = -i + j - 2k
     

     Let's return to the cube with the triangle inside and find the equation of the line segment PB.

     Base Point: P(1,0,0)
     v = PB = -i + j + k
     x = 1 - t
     y = t
     z = t
     

     Now we must place limits on t, since we have a line segment not a full line. At P, t=0, and at B(0,1,1) t=1. So 0<=t<=1.

  2. Equation of a Pane

     

     n is normal to any segment P0P in the plane. Thus the dot product of n and P0P is 0.

     [(x-x0)i + (y-y0)j + (z-z0)k] (dot) (Ai + Bj + Ck) = 0
     A(x-x0) + B(y-y0) + C(z-z0) = 0
     Ax + By + Cz = Ax0 + By0 + Cz0 = D
     
     
     Example:
     A Plane is described by the equation 2x - 3y = 5
     Vector Normal to Plane: 2i - 3j